排序算法Java版,以及各自的复杂度,以及由堆排序产生的top K问题
常用的排序算法包括:
冒泡排序:
每次在无序队列里将相邻两个数依次进行比较,将小数调换到前面, 逐次比较,直至将最大的数移到最后。最将剩下的N-1个数继续比较,将次大数移至倒数第二。依此规律,直至比较结束。时间复杂度:O(n^2)选择排序:
每次在无序队列中“选择”出最大值,放到有序队列的最后,并从无序队列中去除该值(具体实现略有区别)。时间复杂度:O(n^2)直接插入排序:
始终定义第一个元素为有序的,将元素逐个插入到有序排列之中,其特点是要不断的 移动数据,空出一个适当的位置,把待插入的元素放到里面去。时间复杂度:O(n^2)希尔排序:
希尔排序是对直接插入排序的改进,希尔排序是把记录按下标的一定增量分组,对每组使用直接插入排序算法排序;随着增量逐渐减少,每组包含的关键词越来越多,当增量减至1时,整个文件恰被分成一组,算法便终止。快速排序:
递归地将数组一分为2,左边是所有比第一个数小的,右边是所有比第一个数大的。这其中涉及到如何移动交换的技巧问题。本题为挖坑填坑模式。时间复杂度:O(nlogn)堆排序:
对需要排序的数组首先建立堆,然后(如果最终是由小到大排序)依次交换根位置与最后一个节点位置的数值,之后调整堆,循环往复。时间复杂度:O(nlogn)。关于堆这一数据结构以及堆排序可以参考百度百科:堆排序归并排序:给定两个已经排好序(由小到大)的数组,归并排序的意思是,选取数组A中的第一个值,与另一个数组B中的第一个值比较,每找到一个较小的值就写入新的数组C,直到所有的值都大于此值时,将此值写入C,选取数组A的一个值作为对照值,重复上面的操作。时间复杂度:O(nlogn)
关于:各种排序算法时间复杂度和空间复杂度表
关于各种排序算法的稳定性:
1) 稳定的:如果存在多个具有相同排序码的记录,经过排序后,这些记录的相对次序仍然保持不变,则这种排序算法称为稳定的。
插入排序、冒泡排序、归并排序、分配排序(桶式、基数)都是稳定的排序算法。
2) 不稳定的:否则称为不稳定的。
直接选择排序、堆排序、shell排序、快速排序都是不稳定的排序算法。
关于不同引用场景下使用不同的算法:
(1) 若n较小(如n≤50),可采用直接插入或直接选择排序。
当记录规模较小时,直接插入排序较好;否则因为直接选择移动的记录数少于直接插人,应选直接选择排序为宜。
(2) 若文件初始状态基本有序(指正序),则应选用直接插人、冒泡或随机的快速排序为宜;
(3) 若n较大,则应采用时间复杂度为O(nlgn)的排序方法:快速排序、堆排序或归并排序。
快速排序是目前基于比较的内部排序中被认为是最好的方法,当待排序的关键字是随机分布时,快速排序的平均时间最短;
堆排序所需的辅助空间少于快速排序,并且不会出现快速排序可能出现的最坏情况。这两种排序都是不稳定的。
若要求排序稳定,则可选用归并排序。但本章介绍的从单个记录起进行两两归并的 排序算法并不值得提倡,通常可以将它和直接插入排序结合在一起使用。先利用直接插入排序求得较长的有序子文件,然后再两两归并之。因为直接插入排序是稳定 的,所以改进后的归并排序仍是稳定的。
补充:视觉直观感受 7 种常用的排序算法
代码1:冒泡排序,选择排序,直接插入排序,希尔排序,快速排序代码。
1 /**2 * 冒泡排序: 每次在无序队列里将相邻两个数依次进行比较,将小数调换到前面, 逐次比较,直至将最大的数移到最后。
3 * 最将剩下的N-1个数继续比较,将次大数移至倒数第二位。 依此规律,直至比较结束。
4 */
5 public static void bubbleSort(int[] a) {
6 int n = a.length;
7 for (int i = 0; i < n - 1; i++) {
8 for (int j = 0; j < n - i - 1; j++) {
9 if (a[j] > a[j + 1]) {
10 int temp = a[j + 1];
11 a[j + 1] = a[j];
12 a[j] = temp;
13 }
14 }
15 }
16 }
17
18 /**
19 * 选择排序:每次在无序队列中“选择”出最大值,放到有序队列的最后,并从无序队列 中去除该值(具体实现略有区别)。
20 */
21 public static void selectSort(int[] a) {
22 int n = a.length;
23 int max_index = 0;
24 for (int i = n - 1; i >= 0; i--) {
25 max_index = i;
26 for (int j = 0; j <= i; j++) {
27 if (a[j] > a[max_index])
28 max_index = j;
29 }
30 if (max_index != i) {
31 int temp = a[i];
32 a[i] = a[max_index];
33 a[max_index] = temp;
34 }
35 }
36 }
37
38 /**
39 * 插入排序:始终定义第一个元素为有序的,将元素逐个插入到有序排列之中,其特点是要不断的 移动数据,空出一个适当的位置,把待插入的元素放到里面去。
40 */
41 public static void insertSort(int[] a) {
42 int n = a.length;
43 int j = 0;
44 for (int i = 1; i < n; i++) {
45 j = i - 1;
46 int temp = a[i];
47 while (j >= 0 && a[j] > temp) {
48 a[j + 1] = a[j];
49 j--;
50 }
51 a[j + 1] = temp;
52 for (int m : a) {
53 System.out.print(m + ",");
54 }
55 System.out.println();
56 // for (int j = i; j > 0; j--) {
57 // if (a[j] < a[j - 1]) {
58 // int temp = a[j];
59 // a[j] = a[j - 1];
60 // a[j - 1] = temp;
61 // }
62 // }
63 }
64 }
65
66 /**
67 * 快速排序:递归地将数组一分为2,左边是所有比第一个数小的,右边是所有比第一个数大的
68 */
69 public static void quickSort(int[] a) {
70 quickSort(a, 0, a.length - 1);
71 }
72
73 private static void quickSort(int[] a, int l, int r) {
74 if (l < r) {
75 // 取左边第一个值作为分界值
76 int mid = a[l];
77 int i = l, j = r;
78 while (i < j) {
79 while (i < j && a[j] > mid)
80 j--;
81 if (i < j)
82 a[i++] = a[j];
83 while (i < j && a[i] <= mid)
84 i++;
85 if (i < j)
86 a[j--] = a[i];
87 }
88 a[i] = mid;
89 quickSort(a, l, i - 1);
90 quickSort(a, i + 1, r);
91 }
92 }
93
94 /**
95 * 希尔排序是对直接插入排序的改进,希尔排序是把记录按下标的一定增量分组,对每组使用直接插入排序算法排序;随着增量逐渐减少,每组包含的关键词越来越多,
96 * 当增量减至1时,整个文件恰被分成一组,算法便终止。
97 *
98 * @param a
99 */
100 public static void shellSort(int[] a) {
101 int d = a.length;
102 while (true) {
103 d = d / 2;
104 for (int x = 0; x < d; x++) {// 分组
105 // 对每组进行直接插入排序
106 for (int i = x + d; i < a.length; i = i + d) {
107 int temp = a[i];// 记录要插入的值
108 int j;
109 // 将要插入的值插入到正确位置,这一过程中不断检测前面的数,如果相比较大,则同时移动一次
110 for (j = i - d; j >= 0 && a[j] > temp; j = j - d) {
111 a[j + d] = a[j];
112 }
113 a[j + d] = temp;
114 }
115 }
116 if (d == 1) {
117 break;
118 }
119 }
120 }
View Code
代码2:推排序(由于java版比较长,所以另写一个)
1 public class HeapSort { 2 private static int[] sort = new int[] { 1, 0, 10, 20, 3, 5, 6, 4, 9, 8, 12, 17, 34, 11 };
3
4 public static void main(String[] args) {
5 System.out.println(sort.length);
6 buildMaxHeapify(sort);
7 heapSort(sort);
8 print(sort);
9 }
10
11 private static void buildMaxHeapify(int[] data) {
12 // 没有子节点的才需要创建最大堆,从最后一个的父节点开始
13 int startIndex = getParentIndex(data.length - 1);
14 // 从尾端开始创建最大堆,每次都是正确的堆
15 for (int i = startIndex; i >= 0; i--) {
16 maxHeapify(data, data.length, i);
17 }
18 }
19
20 /**
21 * 创建最大堆
22 *
23 * @paramdata
24 * @paramheapSize需要创建最大堆的大小,一般在sort的时候用到,因为最多值放在末尾,末尾就不再归入最大堆了
25 * @paramindex当前需要创建最大堆的位置
26 */
27 private static void maxHeapify(int[] data, int heapSize, int index) {
28 // 当前点与左右子节点比较
29 int left = getChildLeftIndex(index);
30 int right = getChildRightIndex(index);
31
32 int largest = index;
33 if (left < heapSize && data[index] < data[left]) {
34 largest = left;
35 }
36 if (right < heapSize && data[largest] < data[right]) {
37 largest = right;
38 }
39 // 得到最大值后可能需要交换,如果交换了,其子节点可能就不是最大堆了,需要重新调整
40 if (largest != index) {
41 int temp = data[index];
42 data[index] = data[largest];
43 data[largest] = temp;
44 maxHeapify(data, heapSize, largest);
45 }
46 }
47
48 /**
49 * 排序,最大值放在末尾,data虽然是最大堆,在排序后就成了递增的
50 *
51 * @paramdata
52 */
53 private static void heapSort(int[] data) {
54 // 末尾与头交换,交换后调整最大堆
55 for (int i = data.length - 1; i > 0; i--) {
56 int temp = data[0];
57 data[0] = data[i];
58 data[i] = temp;
59 maxHeapify(data, i, 0);
60 }
61 }
62
63 /**
64 * 父节点位置
65 *
66 * @paramcurrent
67 * @return
68 */
69 private static int getParentIndex(int current) {
70 return (current - 1) >> 1;
71 }
72
73 /**
74 * 左子节点position注意括号,加法优先级更高
75 *
76 * @paramcurrent
77 * @return
78 */
79 private static int getChildLeftIndex(int current) {
80 return (current << 1) + 1;
81 }
82
83 /**
84 * 右子节点position
85 *
86 * @paramcurrent
87 * @return
88 */
89 private static int getChildRightIndex(int current) {
90 return (current << 1) + 2;
91 }
92
93 private static void print(int[] data) {
94 int pre = -2;
95 for (int i = 0; i < data.length; i++) {
96 if (pre < (int) getLog(i + 1)) {
97 pre = (int) getLog(i + 1);
98 System.out.println();
99 }
100 System.out.print(data[i] + "|");
101 }
102 }
103
104 /**
105 * 以2为底的对数
106 *
107 * @paramparam
108 * @return
109 */
110 private static double getLog(double param) {
111 return Math.log(param) / Math.log(2);
112 }
113 }
View Code
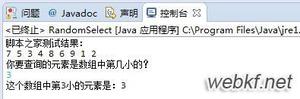
代码3:top-K问题
1 /**2 * 使用最大堆进行top K的选取
3 */
4 public static int[] topK(int[] a, int k) {
5 int[] res = new int[k];
6 if (k > a.length) {
7 System.err.println("k larger than length of array");
8 return res;
9 }
10 for (int i = 0; i < a.length; i++) {
11 if (a[i] > res[0]) {
12 res[0] = a[i];
13 for (int j = 0; j < k && 2 * j + 1 < k;) {
14 int tag1 = 0, tag2 = 0;
15 if (2 * j + 2 < k && res[2 * j + 1] > res[2 * j + 2]) {
16 if (res[j] > res[2 * j + 2]) {
17 int temp = res[2 * j + 2];
18 res[2 * j + 2] = res[j];
19 res[j] = temp;
20 j = 2 * j + 2;
21 tag1 = 1;
22 }
23 } else {
24 if (res[j] > res[2 * j + 1]) {
25 int temp = res[2 * j + 1];
26 res[2 * j + 1] = res[j];
27 res[j] = temp;
28 j = 2 * j + 1;
29 tag2 = 1;
30 }
31 }
32 if (tag1 == 0 && tag2 == 0)
33 break;
34 }
35 }
36 }
37 return res;
38 }
View Code
以上是 排序算法Java版,以及各自的复杂度,以及由堆排序产生的top K问题 的全部内容, 来源链接: utcz.com/z/390725.html