OpenGL ES的多边形三角剖分成三角形带
我正在寻找一种快速的 ,该 可以将不是很复杂的2D凹面多边形(无孔) 分成
准备发送给OpenGL ES进行绘制GL_TRIANGLE_STRIP。
我知道一些算法,但找不到适合我需求的算法:
- http://www.flipcode.com/archives/Efficient_Polygon_Triangulation.shtml
- 该算法可以正常工作,但问题是它返回了无法使用的简单三角形
GL_TRIANGLE_STRIP,需要使用GL_TRIANGLES它,在大量顶点上效率不高。
- 该算法可以正常工作,但问题是它返回了无法使用的简单三角形
- http://code.google.com/p/iphone-glu/
- 它没有任何示例关联,我找不到在OpenGL ES 2.0的iOS上成功使用过它的人
- 我不知道它返回什么,而且好像也调用了我不想要的相应OpenGL命令-我只需要返回三角形
- 它会泄漏内存
我正在开发的平台是:iOS,OpenGL ES 2.0,cocos2d 2.0。
谁能帮我一个这样的算法?或任何其他建议将不胜感激。
回答:
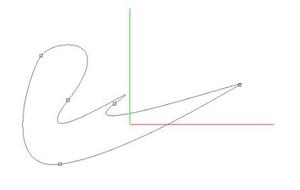
在2D且无孔的情况下,这相当容易。首先,您需要将多边形分解为一个或多个单调多边形。
单调多边形很容易变成三条纹,只需将值排序为y,找到最顶部和最底部的顶点,然后您就可以在左右两边找到顶点列表(因为顶点已定义,顺时针说)。然后,从最顶部的顶点开始,并从左侧和右侧以交替方式添加顶点。
此技术适用于任何不具有自相交边的2D多边形,其中包括某些情况下带有孔的多边形(但必须正确缠绕孔)。
您可以尝试使用以下代码:
glMatrixMode(GL_PROJECTION);glLoadIdentity();
glMatrixMode(GL_MODELVIEW);
glLoadIdentity();
glTranslatef(-.5f, -.5f, 0);
std::vector<Vector2f> my_polygon;
my_polygon.push_back(Vector2f(-0.300475f, 0.862924f));
my_polygon.push_back(Vector2f(0.302850f, 1.265013f));
my_polygon.push_back(Vector2f(0.811164f, 1.437337f));
my_polygon.push_back(Vector2f(1.001188f, 1.071802f));
my_polygon.push_back(Vector2f(0.692399f, 0.936031f));
my_polygon.push_back(Vector2f(0.934679f, 0.622715f));
my_polygon.push_back(Vector2f(0.644893f, 0.408616f));
my_polygon.push_back(Vector2f(0.592637f, 0.753264f));
my_polygon.push_back(Vector2f(0.269596f, 0.278068f));
my_polygon.push_back(Vector2f(0.996437f, -0.092689f));
my_polygon.push_back(Vector2f(0.735154f, -0.338120f));
my_polygon.push_back(Vector2f(0.112827f, 0.079634f));
my_polygon.push_back(Vector2f(-0.167458f, 0.330287f));
my_polygon.push_back(Vector2f(0.008314f, 0.664491f));
my_polygon.push_back(Vector2f(0.393112f, 1.040470f));
// from wiki (http://en.wikipedia.org/wiki/File:Polygon-to-monotone.png)
glEnable(GL_POINT_SMOOTH);
glEnable(GL_LINE_SMOOTH);
glEnable(GL_BLEND);
glBlendFunc(GL_SRC_ALPHA, GL_ONE_MINUS_SRC_ALPHA);
glLineWidth(6);
glColor3f(1, 1, 1);
glBegin(GL_LINE_LOOP);
for(size_t i = 0, n = my_polygon.size(); i < n; ++ i)
glVertex2f(my_polygon[i].x, my_polygon[i].y);
glEnd();
glPointSize(6);
glBegin(GL_POINTS);
for(size_t i = 0, n = my_polygon.size(); i < n; ++ i)
glVertex2f(my_polygon[i].x, my_polygon[i].y);
glEnd();
// draw the original polygon
std::vector<int> working_set;
for(size_t i = 0, n = my_polygon.size(); i < n; ++ i)
working_set.push_back(i);
_ASSERTE(working_set.size() == my_polygon.size());
// add vertex indices to the list (could be done using iota)
std::list<std::vector<int> > monotone_poly_list;
// list of monotone polygons (the output)
glPointSize(14);
glLineWidth(4);
// prepare to draw split points and slice lines
for(;;) {
std::vector<int> sorted_vertex_list;
{
for(size_t i = 0, n = working_set.size(); i < n; ++ i)
sorted_vertex_list.push_back(i);
_ASSERTE(working_set.size() == working_set.size());
// add vertex indices to the list (could be done using iota)
for(;;) {
bool b_change = false;
for(size_t i = 1, n = sorted_vertex_list.size(); i < n; ++ i) {
int a = sorted_vertex_list[i - 1];
int b = sorted_vertex_list[i];
if(my_polygon[working_set[a]].y < my_polygon[working_set[b]].y) {
std::swap(sorted_vertex_list[i - 1], sorted_vertex_list[i]);
b_change = true;
}
}
if(!b_change)
break;
}
// sort vertex indices by the y coordinate
// (note this is using bubblesort to maintain portability
// but it should be done using a better sorting method)
}
// build sorted vertex list
bool b_change = false;
for(size_t i = 0, n = sorted_vertex_list.size(), m = working_set.size(); i < n; ++ i) {
int n_ith = sorted_vertex_list[i];
Vector2f ith = my_polygon[working_set[n_ith]];
Vector2f prev = my_polygon[working_set[(n_ith + m - 1) % m]];
Vector2f next = my_polygon[working_set[(n_ith + 1) % m]];
// get point in the list, and get it's neighbours
// (neighbours are not in sorted list ordering
// but in the original polygon order)
float sidePrev = sign(ith.y - prev.y);
float sideNext = sign(ith.y - next.y);
// calculate which side they lie on
// (sign function gives -1 for negative and 1 for positive argument)
if(sidePrev * sideNext >= 0) { // if they are both on the same side
glColor3f(1, 0, 0);
glBegin(GL_POINTS);
glVertex2f(ith.x, ith.y);
glEnd();
// marks points whose neighbours are both on the same side (split points)
int n_next = -1;
if(sidePrev + sideNext > 0) {
if(i > 0)
n_next = sorted_vertex_list[i - 1];
// get the next vertex above it
} else {
if(i + 1 < n)
n_next = sorted_vertex_list[i + 1];
// get the next vertex below it
}
// this is kind of simplistic, one needs to check if
// a line between n_ith and n_next doesn't exit the polygon
// (but that doesn't happen in the example)
if(n_next != -1) {
glColor3f(0, 1, 0);
glBegin(GL_POINTS);
glVertex2f(my_polygon[working_set[n_next]].x, my_polygon[working_set[n_next]].y);
glEnd();
glBegin(GL_LINES);
glVertex2f(ith.x, ith.y);
glVertex2f(my_polygon[working_set[n_next]].x, my_polygon[working_set[n_next]].y);
glEnd();
std::vector<int> poly, remove_list;
int n_last = n_ith;
if(n_last > n_next)
std::swap(n_last, n_next);
int idx = n_next;
poly.push_back(working_set[idx]); // add n_next
for(idx = (idx + 1) % m; idx != n_last; idx = (idx + 1) % m) {
poly.push_back(working_set[idx]);
// add it to the polygon
remove_list.push_back(idx);
// mark this vertex to be erased from the working set
}
poly.push_back(working_set[idx]); // add n_ith
// build a new monotone polygon by cutting the original one
std::sort(remove_list.begin(), remove_list.end());
for(size_t i = remove_list.size(); i > 0; -- i) {
int n_which = remove_list[i - 1];
working_set.erase(working_set.begin() + n_which);
}
// sort indices of vertices to be removed and remove them
// from the working set (have to do it in reverse order)
monotone_poly_list.push_back(poly);
// add it to the list
b_change = true;
break;
// the polygon was sliced, restart the algorithm, regenerate sorted list and slice again
}
}
}
if(!b_change)
break;
// no moves
}
if(!working_set.empty())
monotone_poly_list.push_back(working_set);
// use the remaining vertices (which the algorithm was unable to slice) as the last polygon
std::list<std::vector<int> >::const_iterator p_mono_poly = monotone_poly_list.begin();
for(; p_mono_poly != monotone_poly_list.end(); ++ p_mono_poly) {
const std::vector<int> &r_mono_poly = *p_mono_poly;
glLineWidth(2);
glColor3f(0, 0, 1);
glBegin(GL_LINE_LOOP);
for(size_t i = 0, n = r_mono_poly.size(); i < n; ++ i)
glVertex2f(my_polygon[r_mono_poly[i]].x, my_polygon[r_mono_poly[i]].y);
glEnd();
glPointSize(2);
glBegin(GL_POINTS);
for(size_t i = 0, n = r_mono_poly.size(); i < n; ++ i)
glVertex2f(my_polygon[r_mono_poly[i]].x, my_polygon[r_mono_poly[i]].y);
glEnd();
// draw the sliced part of the polygon
int n_top = 0;
for(size_t i = 0, n = r_mono_poly.size(); i < n; ++ i) {
if(my_polygon[r_mono_poly[i]].y < my_polygon[r_mono_poly[n_top]].y)
n_top = i;
}
// find the top-most point
glLineWidth(1);
glColor3f(0, 1, 0);
glBegin(GL_LINE_STRIP);
glVertex2f(my_polygon[r_mono_poly[n_top]].x, my_polygon[r_mono_poly[n_top]].y);
for(size_t i = 1, n = r_mono_poly.size(); i <= n; ++ i) {
int n_which = (n_top + ((i & 1)? n - i / 2 : i / 2)) % n;
glVertex2f(my_polygon[r_mono_poly[n_which]].x, my_polygon[r_mono_poly[n_which]].y);
}
glEnd();
// draw as if triangle strip
}
该代码不是最佳代码,但应该易于理解。在开始时,将创建一个凹面多边形。然后创建顶点的“工作集”。在该工作集上,将计算一个排列,该排列按顶点的y坐标对它们进行排序。然后,将该排列循环遍历,以寻找分裂点。一旦找到分割点,就会创建一个新的单调多边形。然后,将新多边形中使用的顶点从工作集中删除,然后重复整个过程。最后,工作集包含无法分割的最后一个多边形。最后,将渲染单调多边形以及三角带顺序。有点混乱,但是我敢肯定您会弄清楚的(这是C
++代码,只需将其放在GLUT窗口中,然后看它能做什么)。
希望这可以帮助 …
以上是 OpenGL ES的多边形三角剖分成三角形带 的全部内容, 来源链接: utcz.com/qa/410628.html